Chapter : 1. Electricity
Combination Of Resistances
Law of Combination of Resistances :
In an electric circuit, the components are connected in several types of combinations. The two different ways called series and parallel combination circuits. In a series combination of the electric circuit, the electric current passes through each of the components of the circuit, and its voltage across the circuit is the sum of the voltages across the component. In the parallel combination of a circuit, the voltage across each component is the same and the total current is the sum of the currents through each component.
Series Combination
When two or more resistances are joined end-to-end so that the same current flows through each of them, they are said to be connected in series.
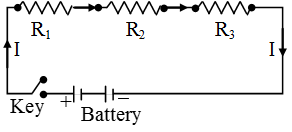
When a series combination of resistances is connected to a battery, the same current (I) flows through each of them.
The Law of a combination of resistances in series:
The law of combination of resistances in series states that when a number of resistances are connected in series, their equivalent resistance is equal to the sum of the individual resistances. Thus, if R1, R2, R3 ..., etc. are combined in series, then the equivalent resistance (R) is given by,
R = R1 + R2 + R3 + ... ....(i)
In the next section of the combination of resistances, we can understand the mathematical derivation of the resistance in series combination and examine the equivalent resistance results. In the combination of resistances, we can use the Ohm’s law and derived the desired formula for the series combination of a particular electrical circuit.
In an electric circuit, the components are connected in several types of combinations. The two different ways called series and parallel combination circuits. In a series combination of the electric circuit, the electric current passes through each of the components of the circuit, and its voltage across the circuit is the sum of the voltages across the component. In the parallel combination of a circuit, the voltage across each component is the same and the total current is the sum of the currents through each component.
Series Combination
When two or more resistances are joined end-to-end so that the same current flows through each of them, they are said to be connected in series.

When a series combination of resistances is connected to a battery, the same current (I) flows through each of them.
The Law of a combination of resistances in series:
The law of combination of resistances in series states that when a number of resistances are connected in series, their equivalent resistance is equal to the sum of the individual resistances. Thus, if R1, R2, R3 ..., etc. are combined in series, then the equivalent resistance (R) is given by,
R = R1 + R2 + R3 + ... ....(i)
In the next section of the combination of resistances, we can understand the mathematical derivation of the resistance in series combination and examine the equivalent resistance results. In the combination of resistances, we can use the Ohm’s law and derived the desired formula for the series combination of a particular electrical circuit.
Trending Articles & Blogs
- Physics Tutor, Math Tutor Improve Your Child’s Knowledge
- How to Get Maximum Marks in Examination Preparation Strategy by Dr. Mukesh Shrimali
- 5 Important Tips To Personal Development Apply In Your Daily Life
- Breaking the Barriers Between High School and Higher Education
- 14 Vocational courses after class 12th
- Tips to Get Maximum Marks in Physics Examination
- Get Full Marks in Biology Class 12 CBSE
Download Old Sample Papers For Class X & XII
Download Practical Solutions of Chemistry and Physics for Class 12 with Solutions
Recent Questions Asked
- Newton’s laws of motion asked by Dr. Mukesh Shrimali
- Process of nutrition in Amoeba asked by Rajiv Sharma
- Importance of studying physics subject in school after 10th asked by Rajiv
- Refraction Through Prism in Different Medium asked by Kirti Sharma
- Ratio and Proportion Question asked by Education Desk
- Explain all the 12 tenses with example asked by Qwerty
- Refraction Through Prism in Different Medium asked by Seema Shrimali